At this point in the learning process, students were encouraged to use math tools—fraction strips, pattern blocks, fraction circles, number lines—any tool that could help verify the equivalency of fractions. Students questioned, "Why do I have to show it if I already know it?"
Good question. But what do the students already "know"? A quick pattern of computation? Or the true conceptual understanding of what it means for fractions to be equivalent? As teachers, we have to be careful not to have students jump right to the abstract. It is important to keep in mind the C-R-A sequence of instruction. That is to start with the concrete (use a math tool to verify reasoning), move to the representational (draw the model pictorially), and then to the abstract (numbers and symbols: reducing, multiplying, etc.) while making connections between the different phases. Check out a previous blog post to read more about C-R-A. Click here.
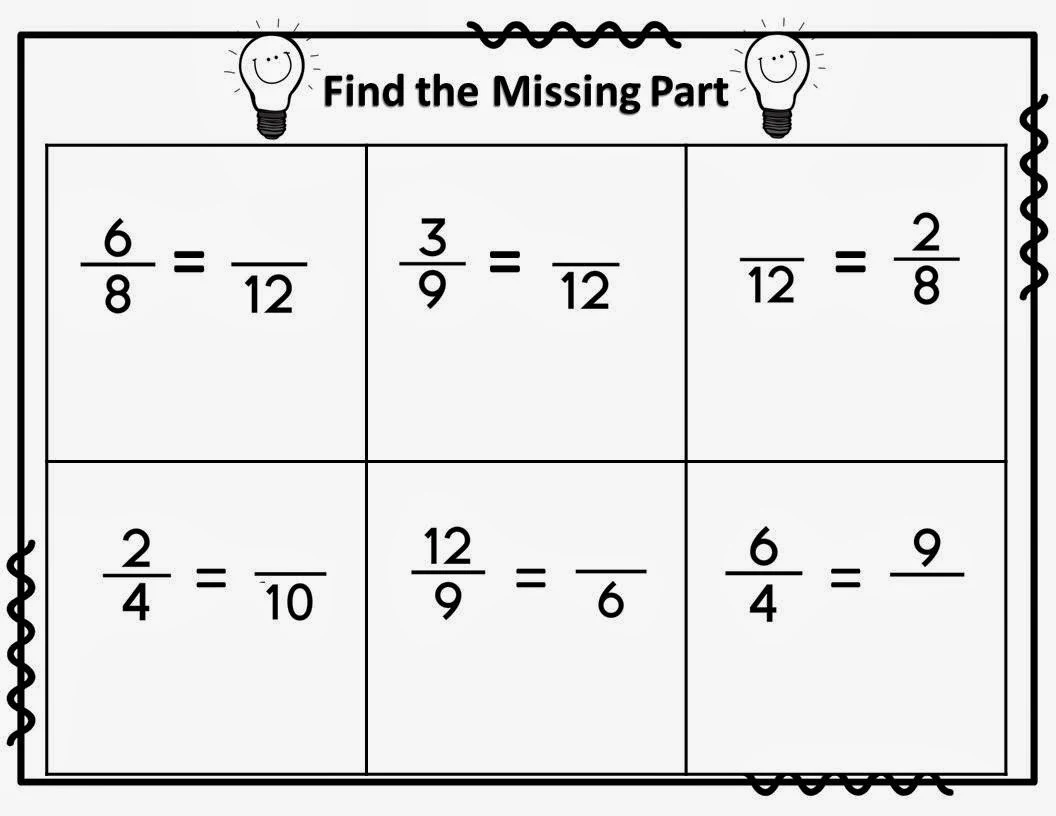
So, we decided to show students fractions where equivalency could not be simply determined by multiplying the numerator and the denominator by the same number. Students would have to reason and verify using the different math tools because the "rule" no longer worked. Check out the fractions below. Can you see how different thinking might be required using problems such as these? Click on the images if you would like to grab a freebie.
 |
When students are working through these types of problems allow them to choose the type of math tool they want to use. Have students see different ways to defend thinking and multiple ways to show the fractions using the different manipulatives.
